
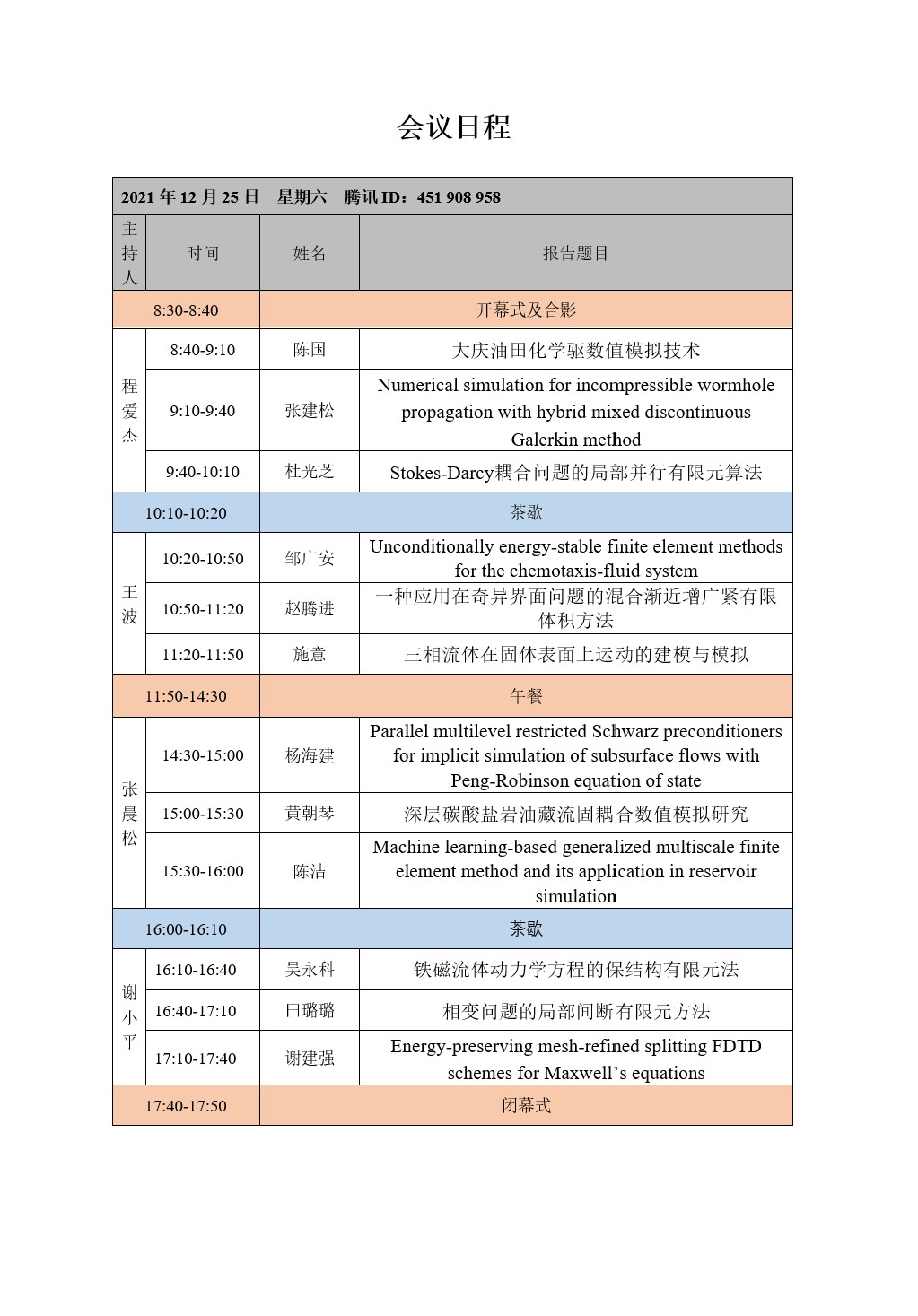
报告内容
大庆油田化学驱数值模拟技术
陈国
单 位:大庆油田有限责任公司勘探开发研究院
摘要:
大庆油田化学驱由于1995年实现了工业化应用,截至2018年,年产油量连续17年保持在1000万吨以上,化学驱产量已占油田年产油的三分之一以上,有力支撑了大庆油田持续高效开发。截至目前,共投注化学驱区块208个,为确保高效开发,方案编制至关重要,需采用数值模拟方法进行开发指标预测、参数优化和效果评价。商业化数值模拟软件满足不了需要,为此,自主研发了化学驱数值模拟软件,具有如下技术特征:
(1)建立了对流弥散扩散基础的油气水三相基本数学模型,实现多种驱动方式一体化模拟和复杂油藏描述;
(2)建立了粘弹性流体驱油机理数学模型,研制了功能先进的新型聚合物驱数值模拟软件;
(3)建立了碱与储层矿物复杂作用驱油机理数学模型,研制了功能完善的三元复合驱数值模拟软件;
(4)发展了化学驱数学模型求解技术,提高了计算速度和精度;
(5)实现化学驱数值模拟并行计算,提高了模拟计算速度;
(6)实现了化学驱数值模拟前后处理一体可视化技术,不仅提高了数值模拟工作效率,而且提升了模拟器工程化水平。
研制的化学驱油数值模拟器取代了引进,已在大庆油田全面推广应用,实现化学驱区块全覆盖。同时,还为国内长庆、国外印尼、苏丹和哈萨克斯坦油田提供技术服务。
Numerical simulation for incompressible wormhole propagation with hybrid mixed discontinuous Galerkin method
张建松
单 位:中国石油大学(华东)
摘要:
In the product enhancement of oil and gas reservoir, wormhole propagation during reactive dissolution of carbonates plays a very important role. A new combined hybrid mixed discontinuous Galerkin (HMDG) finite element method is proposed for solving incompressible wormhole propagation problem. In this method, the hybrid mixed finite element method is established for pressure and velocity equations and the symmetric interior penalty discontinuous Galerkin finite element (IPDGFE) method is constructed for concentration equation, while the porosity function is solved straightly by the given concentration. This combined method not only preserves local mass balance, but also preserves the boundedness of the porosity. The consistency of the method is analyzed and the optimal error estimate is derived. Finally, some numerical results are given to confirm theoretical analysis.
Stokes-Darcy耦合问题的局部并行有限元算法
杜光芝
单 位:山东师范大学
摘要:
报告主要介绍具有BJS交界面条件的Stokes-Darcy耦合问题的局部并行有限元算法,算法可视为Stokes-Darcy耦合问题的两重网格算法和单一问题的局部并行有限元算法的结合。我们首先求解一个粗网格全局耦合问题,将得到的粗网格解作为细网格上交界面项的近似,达到解耦的目的;然后在细网格上结合带有重叠区域的区域分解方法,得到一系列相互独立可并行求解的子问题。为了弥补传统局部并行有限元算法的不足,引入单位分解技巧,利用单位分解函数将细网格上局部解组装为具有全局连续的数值解,并通过增加一步粗网格校正提高速度和测压水头的L2误差收敛阶。
Unconditionally energy-stable finite element methods for the chemotaxis-fluid system
邹广安
单 位:河南大学
摘要:
In this paper, we first derive and analyze a modified Keller-Segel-Navier-Stokes system by introducing a chemotactic stress force, which results from the dissolved oxygen tension, being used to describe the chemotactic movement of bacteria in viscous fluid. Compared with the classical chemotaxis-fluid system, the new system is exactly obeying an energy law. By using the regularization techniques and introducing the auxiliary variable, we carry out the space semi-discretization with the aid of an unconditionally energy-stable finite element method and the time discretization by a backward-Euler scheme. It is proved that, both the semi-discrete (in space) and fully discrete schemes satisfy some discrete energy laws which mimic the differential energy law. The optimal error estimates for both semi-discrete and fully discrete methods, especially for the fluid pressure, are further obtained. Numerical results are also presented to test the accuracy of our proposed schemes and verify the validity of the numerical model.
一种应用在奇异界面问题的混合渐近增广紧有限体积方法
赵腾进
单 位:中原工学院
摘要:
带有界面的非线性奇异微分方程,由于解的奇异性和低正则性,以及界面大跳量比带来的间断问题,为构造高精度的数值计算格式带来很大挑战。基于Puiseux级数对低正则函数的高精确逼近性质,以及渐近分析技巧,通过引入与奇性相关的增广变量,我们构造了一种混合渐近增广紧有限体积格式,数值实验证明了格式的有效性。
三相流体在固体表面上运动的建模与模拟
施意
单 位:山东大学
摘要:
移动接触线问题是指多种不相混溶流体与固体表面接触所形成的问题。众所周知,传统的无滑移边界条件在接触线附近会出现非物理的奇异性。近年来提出的广义Navier边界条件(GNBC)能够较好的解决这一问题,基于其连续模型的计算结果与分子动力学(MD)的结果吻合良好。本工作中,我们将包含广义Navier边界条件的两相流Cahn-Hilliard Navier-Stokes模型推广到三相流动。基于自由能理论,为了满足与两相流模型的相容性条件,三相流模型中的区域内部与边界上的自由能需要进行特别构造。数值算法方面,我们对三相流模型设计了高效的自适应有限元算法。最后,通过气泡穿过两相流界面、复合液滴在固体表面上的运动等数值算例,验证了模型与算法的有效性。
Parallel multilevel restricted Schwarz preconditioners for implicit simulation of subsurface flows with Peng-Robinson equation of state
杨海建
单 位:湖南大学
摘要:
Parallel algorithms and simulators with good scalabilities are particularly important for large-scale reservoir simulations on modern supercomputers with a large number of processors. In this talk, we introduce and study a family of highly scalable multilevel restricted additive Schwarz (RAS) methods for the fully implicit solution of subsurface flows with Peng-Robinson equation of state in two and three dimensions. With the use of a second-order fully implicit scheme, the proposed simulator is unconditionally stable with the relaxation of the time step size by the stability condition. The investigation then focuses on the development of several types of multilevel overlapping additive Schwarz methods for the preconditioning of the resultant linear system arising from the inexact Newton iteration, and some fast solver technologies are presented for the assurance of the multilevel approach efficiency and scalability. We numerically show that the proposed fully implicit framework is highly efficient for solving both standard benchmarks as well as realistic problems with several hundreds of millions of unknowns and scalable to 8192 processors on the Tianhe-2 supercomputer.
深层碳酸盐岩油藏流固耦合数值模拟研究
黄朝琴、刘礼军、张旭、姚军
单 位:中国石油大学(华东)
摘要:
我国碳酸盐岩油气资源丰富,埋藏深(> 4500 m),是增储上产的重要领域。碳酸盐岩储层岩石孔隙类型多样,包括基质孔隙、溶蚀孔洞和裂缝,非均质性极强,储集空间类型跨越多个尺度,宏观上表现为渗流-自由流耦合特征;且埋藏较深,裂缝和溶蚀孔洞在开发过程中易发生变形,具有强应力敏感性。对此,考虑储层介质的弹性变形,建立了介观尺度上的离散缝洞模型的流固耦合数学模型及其数值模拟方法。其中,多孔介质渗流区域采用Biot方程,在渗流场和应力场中均对裂缝进行降维处理,建立了离散裂缝的Biot流固耦合模型;溶洞为自由流区域,采用Navier-Stokes方程;两个区域间通过扩展的Beavers-Joseph-Saffman条件进行耦合。应用混合有限元方法对该流固耦合模型进行了数值求解,其中,渗流区域采用经典的Galerkin有限元方法,自由流区域采用Taylor-Hood混合元方法,通过数值算例验证了模型和方法的正确性。在此基础上,基于离散缝洞模型应用均匀化理论推导建立了宏观尺度流固耦合数学模型,采用有限元方法分别求解2个固体变形元胞问题和1个流体流动元胞问题,形成了储层岩石的等效弹性模量、Biot有效应力系数、渗透率等参数的计算方法和技术。
Machine learning-based generalized multiscale finite element method and its application in reservoir simulation
陈洁
单 位:西交利物浦
摘要:
In multiscale modeling of subsurface fluid flow in heterogeneous porous media, standard polynomial basis functions are replaced by multiscale basis functions to acquire multiscale properties. To produce such functions, a number of Partial Differential Equations (PDEs) must be solved, so it makes sense to replace PDEs solvers with data-driven methods, given their great capabilities and general acceptance in recent decades. A mixed Generalized Multiscale Finite Element Method (mixed GMsFEM) has been recently proposed for subsurface flow problems, which approximates the pressure and velocity in the multiscale and fine grid space, respectively. The main purpose of this talk is to develop four distinct Convolutional Neural Network (CNN) models to predict four different multiscale basis functions for the mixed GMsFEM. These models have been applied to the 249,375 samples generated by the MatLab software, with the permeability field as the only input. The statistical results indicate that the four developed models yield satisfactory outputs with a coefficient of determination (R2) of 0.8328 - 0.9049 and Mean Squared Error (MSE) of 0.0109 - 0.0261. Graphically, all models follow the observed trend in each coarse block. Looking at this work as an image (matrix)-to-image (matrix) regression problem, the constructed deep learning-based models may have applications beyond reservoir engineering, such as hydrogeology and rock mechanics.
铁磁流体动力学方程的保结构有限元法
吴永科(电子科技大学) 谢小平 (四川大学)
摘要:
铁磁流体动力学方程刻画了铁磁流体在外加磁场作用下的动力学行为,由静磁场方程、Navier-Stokes方程和磁化方程通过开尔文力耦合而成。在本报告中,将首先介绍连续方程的能量不等式;而后构造有限元离散,使得连续方程的能量不等式在离散情况下得到自然保持;最后,将讨论有限元方程解的存在性以及最优误差估计。我们将展示系列数值算例,来验证理论结果的正确性。
相变问题的局部间断有限元方法
田璐璐
单 位:中国石油大学(华东)
摘要:
We present a local discontinuous Galerkin (LDG) method for a hyperbolic-elliptic system modeling the propagation of phase transition in solids and fluids. The physical and mathematical difficulties of the phase transition models are discussed first. Then we give the LDG discretization and the L − 2 stability of the numerical scheme. When the stress-strain relation of the phase transition model is linear, we provide an error estimate for the LDG discretization assuming the exact solution is sufficiently smooth. Finally, numerical experiments are performed to verify the accuracy of the LDG discretization and to solve Riemann problems containing both phase transition and shock waves. The extension of the LDG discretization to Navier-stokes-Korteweg(NSK) equations modeling phase transition in fluid dynamics are introduced in the end.
Energy-preserving mesh-refined splitting FDTD schemes
for Maxwell’s equations
谢建强
单 位:安徽大学
摘要:
In this talk, we develop and analyze two types of energy-preserving local mesh-refined splitting finite difference time-domain (EP-LMR-S-FDTD) schemes for Maxwell’s equations. For the local mesh refinements, it is challenging to define the suitable local interface schemes which can preserve energy and guarantee high accuracy. We propose the efficient local interface schemes and fast implementation of the corresponding schemes.We further prove the convergence of the schemes and obtain the error estimates. Numerical experiments are given to show the performance of the EP-LMR-S-FDTD schemes which confirm theoretical results. This is joint work with Prof. Zhiyue Zhang (Nanjing Normal Unviersity), and Prof. Dong Liang (York University,Canada).